Mathematics Grade 12 Questions and AnswersTrigonometry Questions and Answers Mathematics Grade 12 Pdf Download

Trigonometry Questions and Answers Mathematics Grade 12 Pdf Download

Trigonometry Questions and Answers Mathematics Grade 12 Pdf Download. Trigonometry is a branch of mathematics that focuses on relationships between the sides and angles of triangles. The word trigonometry comes from the Latin derivative of Greek words for triangle (trigonon) and measure (metron).

TRIGONOMETRY QUESTIONS AND ANSWERS GRADE 12

Activity 1

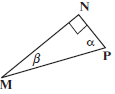
  1. ∆ MNP is a right-angled triangle. Write down the trig ratios for:
    1. sin α
    2. sin β (4)
    3. tan β
  2. cos α (3)
  3. If MP = 13 and NP = 5, calculate cos β.
    6
    [7]
Solutions

    1. sin α = MN(1)
      MP
    2. sin β = NP(1)
      MP
    3. tan β = NP(1)
      MN
    4. cos α = NP(1)
      MP(4)
  2. MP = 13 and NP = 5, so we can find MP,
    MP2 = MN2 + NP2 ……….Pythagoras
    132 = MN2 + 52
    169 = MN2 + 25
    MN2 = 169 – 25
    MN2 = 144
    ∴MN = 12
    cos β = MN = 12
    MP    13(3)
    [7] 

Activity 2

  1. If sin θ is negative and cos θ is positive, then which statement is true?
    1. 0° < θ < 90°
    2. 90° < θ < 180°
    3. 180° < θ < 270°
    4. 270° < θ < 360° (1)
  2. If tan θ < 0 and cos θ < 0, then which statement is true?
    1. 0° < θ < 90°
    2. 90° < θ < 180°
    3. 180° < θ < 270°
    4. 270° < θ < 360° (1)
  3. Will the following trig ratios be positive or negative?
    1. sin 315°
    2. cos (–215°)
    3. tan 215°
    4. cos 390° (4)
      [6]
Solutions

  1. Sin θ is negative in 3rd and 4th quadrants; cos θ is positive in 1st and 4th quadrants.
    So θ is in the 4th quadrant. D. 270° < θ < 360° 3 (1)
  2. tan θ < 0 in 2nd and 4th quadrants; cos θ < 0 in 2nd and 3rd quadrants.
    So θ is in the 2nd quadrant. B. 90° < θ < 180° 3 (1)
    1. sin 315° is in 4th quadrant so it is negative. 3 (1)
    2. cos (–215°) is in 2nd quadrant so it is negative. 3 (1)
    3. tan 215° is in 3rd quadrant, so it is positive. 3 (1)
    4. cos 390° is the same as cos 30° in the 1st quadrant, so it is positive. 3 (1)
      [6]

Activity 3
If cos β = p/√5 where p < 0 and β ∈ [180°; 360°], determine, using a diagram, an expression in terms of p for:

  1. tan β
  2. 2 cos2β – 1
    [6]
Solutions

  1. cos β =p/√5 = x/r = ; so x = p and r = √5
    By Pythagoras, y2 = r2 – x2
    ∴ y2 = (√5)2 – p2
    = 5 – p2
    ∴ y = ± √5 – p2
    ∴ y = – √5 – p2 since β is in quadrant 3, y is negative
    ∴ tan β = –√5 – p2 (4)
    p
    11
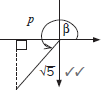  2. 2 cos2β – 1 = 2 ( p/√5)2 – 1
    2p 2 -1 (2) [6]
    5

Activity 4
Without using a calculator, determine the value of:

  1. cos 150°
  2. sin (–45°)
  3. tan 480°
    [7]
Solutions

  1. cos 150° rewrite as (180 – ?)
    = cos(180° – 30°) quadrant II, cos θ negative
    = –cos 30° special ratios
    = –√3/2 (2)
  2. sin(–45°) sin(–θ) = –sin θ; quadrant IV, sin θ negative
    = –sin 45° special ratios
    = – 1/√2 (2)
  3. tan 480° write as an angle in the first rotation of 360°
    = tan (480° – 360°)
    = tan 120°  quadrant II, rewrite as (180 – ?)
    = tan (180° – 60°) tan θ negative
    = –tan 60° special ratios
    = –√3  (3)
    [7] 

Activity 5
Write the trig ratios as the trig ratios of their co-functions:

  1. sin 50°
  2. cos 70°
  3. sin 100°
  4. cos 140°
    [4]
Solutions

  1. sin 50° = sin(90° – 40°) = cos 40°
  2. cos 70° = cos(90° – 20°) = sin 20°
  3. sin 100° = sin(90° + 10) = cos 10°
  4. cos 140° = cos(90° + 50°) = –sin 50°
    [4] 

Summary
Any angle (obtuse or reflex) can be reduced to an acute angle by using:

  • Convert negative angles to positive angles
  • Reduce angles greater than 360°
  • Use reduction formulae
  • Use co-functions

Activity 6
Simplify without using a calculator:

  1. sin(180° + x). cos 330°.tan 150° (4)
    sin x
  2.       cos 750°.tan 315°.cos(–θ)      (8)
    cos(360°- θ).sin300°.sin(180°- θ)
  3. tan 480°.sin 300°.cos 14°.sin(–135°) (9)
    sin104°.cos225°
  4.     cos 260°.cos 170°   (7)
    sin10°.sin190°.cos350°
    [28]

20

Activity 7
Simplify the following expressions.

  1.      cos (180°–x) sin (x –90°) – 1    (8)
    tan2(540° + x) sin(90°+x)cos(–x)
  2. [sin(–θ) + cos(360° + θ)][cos(θ – 90°) + cos(180°+θ)] (3)
  3. cos2θ (1 + tan2θ) (3)
  4. 1 – cos2θ
    1– sin2θ (3)
    [17]
Solutions

  1.     cos (180°–x) sin (x –90°) – 1    (8)
    tan2(540° + x) sin(90°+x)cos(–x)  – use reduction formulae and co-functions
    =         (–cos x)(–cos x) –1
    tan2(540°- 360°+ x) cos x. cos x
    – multiply out numerator and denominator reduction of angle > 360°
    =        cos2x–1
    tan2(180°+ x).cos2x
    – use trig identity format for cos2x – 1 reduction formula
    =    –(1 – cos2x)
    tan2 x . cos2x
    – use trig identities for 1 – cos2x and for tan x
    =   –sin2x
    sin2x . cos2x
    cos2x     1
    = sin2x = -1
    sin2x
    – simplify
    (8)
  2. [sin(–θ) + cos(360° + θ)][cos(θ – 90°) + cos(180°+θ)] – reduce to angle < 90°
    =[–sin θ + cos θ][cos (–(90° – θ))+ (–cos θ)] – simplify; use co-functions
    =(–sin θ + cos θ)(sin θ – cos θ) – multiply out using FOIL
    = –sin2 θ + sin θ cos θ + cos θ sin θ – cos2 θ
    = –(sin2θ + cos2θ) + 2 sin θ cos θ – use trig identity
    = –1 + 2 sin θ cos θ – use double angle identity
    = –1 + sin2θ (3)
  3. cos2θ (1 + tan2θ) – multiply out the bracket
    =cos2θ + cos2θ.tan2θ – use trig identity for tan θ
    = cos2θ + cos2θ . sin2θ – simplify
    1        cos2θ.
    = cos2θ + sin2θ 3 = 1 – use trig identity sin2θ + cos2θ = 1 (3)
  4. 1 – cos2θ. – use trig identity sin2θ + cos2θ = 1
    1 – sin2θ
    = sin2θ – use trig identity for tan θ
    cos2θ
    = tan²θ (3)
    [17]

Activity 8
Prove the following identities:

sin x ∙tan x + cos x =   1
cos x (4)

(sin x + tan x) (   sin x   ) = sin x. tan x (7)
1 + cos x

1     =   cos x   + tan x (6)
cos x    1 + sin x

1   + tan x = tanx  (5)
tanx                sin2x
[22]

22
23

Hints for solving trig identities:

  • Choose either the lefthand side or the righthand side and simplify it to look like the other side.
  • If both sides look difficult, you can try to simplify on both sides until you reach a point where both sides are the same.
  • It is usually helpful to write tan θ as sinθ
    cosθ .
  • Sometimes you need to simplify sinθ  to tan θ.
    cos θ
  • If you have sin2x or cos2x with +1 or –1, use the squares identities (sin2θ + cos2θ = 1).
  • Find a common denominator when fractions are added or subtracted.
  • Factorise if necessary – specify with examples i.e. common factor, DOPS, Trinomial, sum/diff of two cubes

Activity 9

  1. If cos 20° = p, determine the following ratios in terms of p:
    1. cos 380°
    2. sin 110°
    3. sin 200° (6)
  2. Determine the general solution for x in the following equations:
    1. 5 sin x = cos 320° (correct to 2 decimal places)
    2. 3 tan x + √3 = 0 (without using a calculator)
    3. tan x–1 = –3 (correct to one decimal place) (10)
      2
  3. Determine x for x ∈[–180°; 180°] if 2 + cos (2x – 10°) = 2,537 (6)
    [22]
Solutions

  1. cos 20° = p/1so x = p and r = 1
    By Pythagoras, y2 = r2 – x2
    y2 = 12 – p2 = 1– p2
    y = √1 – p2 first quadrant, so y is positive

    1. cos 380° = cos (360° + 20°) = cos 20°  = p  (2)
    2. sin 110° reduction formula
      = sin (180° – 70°)
      = sin 70° 3 co-function
      = sin (90° – 20°)
      = cos 20° 3 = p 3 (3)
    3. sin 200° = sin (180° + 20°)
      = –sin20°
      = – √1 – p2 = –√1 – p2   (1) (6)
    1. 5 sin x = cos 320°
      5 sin x = 0,766044
      sin x = 0,15320…
      Ref angle = 8,81°
      x = 8,81° + k360° OR x = 180° – 8,81° + k360°
      x = 171,19° + k360°  k ∈ Z (4)
      Calculator keys:
      cos 320 =
      ÷ 5 =
      SHIFT sin ANS =
    2. 3 tan x + √3 = 0
      3 tan x = – √3
      tan x = – √3/3 [special angle: tan 30° tan 30° = – √3/3]
      Ref angle = 30°
      x = 180° – 30° + k180°
      x = 150° + k180° 3 k ∈ Z (3)
    3. tan x–1 = –3 multiply both sides by 2
      2
      tan x – 1 = –6
      tan x = –5 reference angle is 78,69…°
      ∴x = 180° –78,69…° + k180°
      x = 101,31° + k180°; k ∈ Z (3) (10)
  3. 2 + cos (2x – 10°) = 2,537
    cos (2x – 10°) = 0,537
    Ref angle = 57,52…..°
    2x – 10° = 57,52…..° + k360° or 2x – 10° = 360° – 57,52° + k360°
    [solve equations]
    2x = 67,52….° + k360° or 2x = 312,48…° + k360°
    [divide all terms on both sides by 2]
    x = 33,76° + k180° or x = 156,24° + k180° 3 k ∈ Z
    x ∈ [–180°; 180°]
    So for k = –1: x = 33,76° –180° = –146,24° or x = 156,24° – 180° = –23,76°
    For k = 0: x = 33,76° or x = 156,24°
    (For k = 1, x will be > 180°, so it is too big)
    Solution: x ∈ {–146,24°; –28,76°; 33,76°; 156,24°}  (6)
    [22] 

Activity 10
Do NOT use a calculator to answer this question. Show ALL calculations. Prove that:

  1. cos 75° =√2 ( √3 –1) (5)
    4
  2. Prove that cos(90° – 2x).tan(180° + x) + sin2(360° – x) = 3sin2x (7)
  3. Prove that (tan x – 1)(sin 2x – 2cos2x) = 2(1 – 2sin x cos x) (7)
    [19]
  1. Solutions
    1. LHS = cos 75° = cos(45° + 30°)
    = cos45°.cos30° – sin45°.sin30°
    = √2/2 . √3/√2 – √2/2 . ½
    = √2 . √3 – √2/4
    4
    =√2 (√3 –1 = RHS (5)
    4
  2. LHS = cos(90° – 2x).tan(180° + x) + sin2(360° – x) co-functions and reductions
    = sin2 x. tan x + sin2 x                                               double angle for sin 2x
    trig identity for tan x
    = 2sin x.cos x. sin x + sin2 x simplify
    cos x
    = 2 sin2 x + sin2 x
    = 3 sin2x = RHS (7)
  3. There are several ways to prove this. Here is one solution.
    LHS = (tan x – 1)(sin 2x – 2cos2x)
    = (sin x – 1 ) (2sin x. cos x – 2cos2x) double angle identity for sin 2x
    cos x
    = 2sin2 x – 2sin x. cos x – 2sin x. cos x + 2cos2x multiply out
    = 2 sin2 x – 4 sin x cos x + 2 cos2 x
    = 2(sin2 x – 2sin x. cos x + cos2x)              trig identity sin2 x + cos2 x = 1
    = 2(1 – 2sin x. cos x) = RHS (7)
    [19]

Activity 11
Determine the general solution for x in the following:

  1. sin 2x. cos 10° – cos 2x. sin 10° = cos 3x (8)
  2. cos2 x = 3 sin 2x (11)
  3. 2sinx = sin(x + 30°) (5)
    [24]
Solutions

  1. sin 2x. cos 10° – cos 2x. sin 10° = cos 3x use compound angle identity
    ∴ sin (2x – 10°) = cos 3x use co-functions
    ∴ sin (2x – 10°) = sin (90° – 3x)
    ∴ 2x – 10° = 90°–3x + k360°3or 2x–10° = 180°–(90°–3x) + k360°3 k ∈ Z
    ∴ 5x = 100° + k360° 2x – 10° = 90° + 3x + k360°
    ∴ x = 20° + k72° –x = 100 + k360°3
    x = –100 – k360 3 k ∈ Z (8)
  2. cos2 x = 3 sin 2x use double angles for sin 2x
    cos2 x = 3(2 sin x.cos x)3 make LHS = 0
    cos2 x – 3(2 sin x.cos x) = 0 multiply out
    cos2 x – 6 sin x.cos x = 0 common factor
    cos x (cos x – 6 sin x) = 0
    ∴ cos x = 03 or cos x – 6 sin x = 0
    cos x = 0 or cos x  = 6sin x
    cos x      cos x
    cos x = 0 or 1 = 6 tan x
    cos x = 0 or tan x = 1/6
    Reference angle = 90° or reference angle = 9,46°
    ∴ x = 90° + k360° or x = 360°–90° + k360° or x = 9,46° + k180° 3k ∈ Z
    x = 270° + k360° or x = 180° + 9,46° + k360°  k ∈ Z
    = 189,46° + k360°  k ∈ Z (11)
  3. 2 sin x = sin ( x + 30° )
    2 sin x = sin x. cos30° + cosx.sin30°3
    2 sin x = sin x. √3/2  + cos x. ½ multiply by 2
    4 sin x = √3 sin x + cos x divide by cos x
    4 tan x = √3 tan x + 1
    4 tan x − √3 tan x = 1
    tan x =    1
    4 − √3
    x = 23,79° + k180°; k ∈ Z 3 (5)
    [24] 

 

Related:

© www.myexampapers.com. All rights reserved.