Mathematics Grade 12 Questions and AnswersCalculus Questions and Answers Mathematics Grade 12 Pdf Download

Calculus Questions and Answers Mathematics Grade 12 Pdf Download

Calculus is the mathematical study of change, in the same way that geometry is the study of shape and algebra is the study of operations and their application to solving equations. Calculus Questions and Answers Mathematics Grade 12 Pdf Download

CALCULUS QUESTIONS AND ANSWERS GRADE 12

Activity 1

    1. Determine the average gradient of the graph of y = 5x2 – 4 between x = –4 and x = –1
    2. Is the function increasing or decreasing between x = –4 and x = –1? (3)
  2. Determine the average gradient of the graph of y = 5x2 –4 between:
    1. x = 1 and x = 3
    2. x = 2 and x = 3
    3. x = 2,5 and x = 3
    4. x = 2,99 and x = 3 (8)
    1. Calculate the average gradient of the curve f(x) = x(x + 3) between x = 5 and x = 3.
    2. What can you deduce about the function f between x = 5 and x = 3? (3)
      [14]
Solutions

    1. At x = –4
      y = 5(–4)2 – 4 = 80 – 4 = 76
      At x = –1
      y = 5(–1)2 – 4 = 5 – 4 = 1
      y2 − y1 = 76 − 1 = 75 = − 25 (2)
      x2 – x1     -4-(-1)    -3
    2. The function is decreasing between x = –4 and x = –1 because the gradient is negative.(1)
    1. The points at x = 1 and x = 3 are (1; 1) and (3; 41)
      m = y2 − y1 = 41 − 1 = 40 = − 20 (2)
      x2 – x1     3 – 1       2
    2. The points at x = 2 and x = 3 are (2; 16) and (3; 41)
      m = y2 − y1 = 41 − 16 = 25 = 25 (2)
      x2 – x1      3 – 2        1
    3. The points at x = 2,5 and x = 3 are (2,5; 27,25) and (3; 41)
      m = y2 − y1 = 41 − 27.25 = 13.75 = 27.5 (2)
      x2 – x2        3 -2.5           0.5
    4. The points at x = 2,99 and x = 3 are (2,99; 40,7) and (3; 41)
      m = y2 − y1 = 41 − 40.7 = 0.3 = 30 (2)
      x2 – x1       3 – 2.99    0.01
    1. The points are (5; 40) and (3; 18).
      m = y2 − y1 = 18 − 40 = − 22 = 11 (2)
      x2 – x1        3 – 5         -2
    2. The function is increasing between x = 5 and x = 3. (1)
      [14] 

Use the equation of the curve y = 5x2 –4 to calculate the y-values.
Use the y2 − y1 formula to calculate the gradient.
x2 – x1

Calculate the gradient of a curve?
1

  • The average gradient between two points on a curve is equal to the gradient of the straight line through the points. So the average gradient of curve AB is 12.
  • As the two points are moved closer together, the average gradient approaches the gradient of the curve which is also the gradient of the tangent to the curve at that point. So the gradient of the curve AB at point B is 30.
  • Remember that the tangent is a line that touches a curve at one point only.
  • The average gradient tells us whether the graph is increasing or decreasing between those points.
  • If the function is decreasing between two points, the average gradient will be negative.
  • If the function is increasing between two points, the average gradient will be positive.

Activity 2

  1. Determine f ´(x) from first principles if f(x) = 5x2 – 4x + 2 (6)
  2. Determine f ´(x) from first principles if f(x) = 2/x (6)
    [12]
Solutions

  1. f(x + h) = 5(x + h)2 − 4(x + h) + 2
    = 5(x2 + 2xh + h2 ) − 4x − 4h + 2
    = 5x2 + 10xh + 5h2 − 4x − 4h + 2
    f ´(x) =  lim f(x + h) − f(x) =  lim 5x2  + 10xh +  5h2  − 4x − 4h + 2 − ( 5x2  − 4x + 2)
    h➞0         h             h➞0                                     h
    = lim 10xh +  5h2  − 4h
    h➞0          h
    = lim h(10x + 5h − 4)
    h➞0          h
    = lim (10x + 5h − 4)
    h➞0
    = 10x – 4 (6)
  2. f(x + h) =    2
    x + h
    3

(6)
[12]

Activity 3

  1. Evaluate Dx[ (x3 – 3)2]
  2. Find f ´(x) if f(x)= 3√x
  3. Find d 3√x5
    dx
  4. Differentiate f(x) if f(x) = √x4
  5. Find f ´(x) if f(x) = √16x3
    [11]

4Rewrite terms which are ‘fractions’ where x is part of the denominator, 1/xn as x−n so that you can use the rule: if f(x) = xn then f ´(x) = nxn–1
e.g.6
Find f ´(x) if f(x) = 3x 2
4x3

Solution
f(x) = 3x2 = 3 x−1
4x3     4
So f ´(x) = − 3 x−2 = − 3
4            4x2

Activity 4

  1. Determine, using the rules of differentiation: dy  if y = √x –  1  (3)
    dx            2     6x3
  2. Evaluate dy if y = 4 –  x 3
    dx         √x   9   (3)
  3. Determine Dx [ 6x + 5 ] (4)
    3x2
    [10]
Solutions

  1. y = √x −  1
    2     6x3
    y = ½x½ – 1/6 x-3 First rewrite the terms in the form kxn
    dy = ¼x-½ + 3/6x-4 Use the rules of differentiation
    dx
    dy = ¼x-½ + ½x-4 Simplify
    dx
    dy = 1/4√x + ½x-4 Change back to surds and positive exponents(3)
  2. y = 4 − x 3
    √x    9
    y = 4x-½ – 1/9x-3 First rewrite the terms in the form kxn
    dy  = -½ . 4(x-½-1) – 3.1/9x2 Use the rules of differentiation
    dx
    5
    (4)
    [10]

Activity 5

  1. f(x) = − x3 − x2 + x + 10
    1. Write down the coordinates of the y-intercept of f
    2. Show that (2; 0) is the only x-intercept.
    3. Calculate the coordinates of the turning points of f
    4. Sketch the graph of f. Show all intercepts with axes and all turning points.
    5. Determine the point of inflection. (17)
  2. Sketched below is the graph of g(x) = –2x3 – 3x2 + 12x + 20 = –(2x – 5)(x + 2)2. A and T are turning points of g. A and B are the x-intercepts of g. P(–3; 11) is a point on the graph.
    9

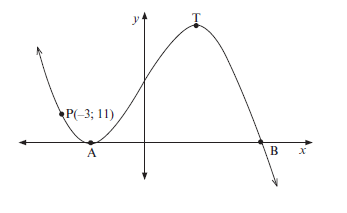
    1. Determine the x-coordinate of T.
    2. Determine the equation of the tangent to g at P(–3; 11) in the form y = …
    3. Determine the value(s) of k for which –2x3 – 3x2 + 12x + 20 = k has three distinct roots.
    4. Determine the x-coordinate of the point of inflection. (14)
      [31]
Solutions

    1. When x = 0, y = 10, therefore are (0; 10) 3 (1)
    2. Assuming that (2; 0) is the x-intercept, then x –2 is a factor of f(x)
      f(x) = − x3 − x2 + x + 10 = (x − 2)( − x2 − 3x − 5)
      ∴ x − 2 = 0 or − x2 − 3x − = 0
      x = 2 but − − x2 − 3x − 5 = 0 has no real solution. Hence (x – 2) is the only x-intercept (5)
    3. At the turning point f ´(x) = − 3x2 − 2x + 1 = 0
      (–3x + 1)(x +1) = 0
      x = 1/3 or x = – 1
      When x = 1/3 ,y = -1/27 – 1/9 + 1/3 + 10 = 270 – 3 + 9 + 1 = 275 = 105/27
      27                27
      Therefore turning point is (1;9)
    4. 10(4)
    5. At the point of inflection f ´´(x) =  − 6x − 2 = 0
      ∴ at x = -2/6 = -1/3
    1. Since A and B are the x-intercepts of g they are solutions of –(2x – 5)(x + 2)2 = 0
      i.e. x = –2 and x = 5/2 The distance between –2 and  is 5/2  is 5/2-(-2) = 4,5 units (2)
    2. T is a turning point. g´(x) = –6x2 –6x + 12 = 0.
      –6(x2 + x – 2) = 0
      –6(x + 2)(x – 1) = 0
      When x = –2 or x = 1.
      So the x-coordinate of T is 1. (3)
    3. g´(3) = –6(–3)2 –6(–3) + 12 = –24
      So the equation of the tangent line is y – 11 = –24(x + 3) which simplifies to y = –24x – 61 (3)
    4. The graph of y = k is shown together with g(x) below.
      Using these graphs we can observe that, provided the line lies above the y-value of A and below that of T, the equation
      –2x3 – 3x2 + 12x + 20 = k will have 3 distinct roots.
      At T, g(1) = –2 – 3 + 12 + 20 = 27. So for 0 < k < 27 the equation has 3 distinct roots.
      11
      (4)
    5. g´´(x) = –12x – 6
      –12x – 6 = 0 when x = 6/-12 = -½ (2)
      [31] 

Activity 6

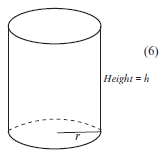
  1. A drinking glass, in the shape of a cylinder (shown here), must hold 200 ml of liquid when full.
    Find the value of r for which the total surface area of the glass is a minimum.
  2. A rectangular box is constructed in such a way that the length (l) of the base is three times as long as its width. The material used to construct the top and the bottom of the box costs R100 per square metre. The material used to construct the sides of the box costs R50 per square metre. The box must have a volume of 9 m3. Let the width of the box be x metres.
    26
    2.1 Determine an expression for the height (h) of the box in terms of x.
    2.2 Show that the cost to construct the box can be expressed as
    C = 1200 + 600x2
    x
    27
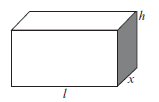
    2.3 Calculate the width of the box (that is the value of x) if the cost is to be a minimum.
  3. A tourist travels in a car over a mountainous pass during his trip.
    The height above sea level of the car, after t minutes, is given as s(t) = 5t3 − 65t2 + 200t + 100 metres. The journey lasts 8 minutes.
    3.1 How high is the car above sea level when it starts its journey on the mountainous pass?
    3.2 Calculate the car’s rate of change of height above sea level with respect to time, 4 minutes after starting the journey on the mountainous pass.
    3.3 Interpret your answer to QUESTION 3.2.
    3.4 How many minutes after the journey has started will the rate of change of height with respect to time be a minimum? (3)
    [26]
Solutions

  1. Find an equation for what you want to minimise:
    Surface area of glass = area of base + area of curved surface
    So S = πr2 + 2πrh
    Because you cannot take the derivative if there are two different variables in the equation (r and h), you must use other information to help you get the equation for what you want to minimise in terms of one variable only.
    We know the glass holds 200 ml = 200 cm3.
    The volume of the glass is πr2h
    So πr2h = 200 so h = 200
    πr2
    And so we can say
    S = πr2 + 2πr ( 200/πr2) = πr2 + 400/r)
    Now the only variable is r, because π is a constant.
    Write S in a way that is easy to find the derivative:
    S = πr2 + 400r−1
    Take the derivative of the function you want to minimise:
    S´ = 2πr − 400r−2
    Put the derivative equal to 0:
    2πr − 400r−2 = 0
    2πr = 400r−2
    2πr3 = 400 r ≠ 0
    r3 =  400
    2π
    so r = 3 √400/2π ≈ 3.99 cm  (6)
  2. 2.1 Volume = l × b × h
    9 = 3x.x.h
    9 = 3x2h
    h = 3/x2  (3)
    2.2 C = [ 2(3xh) + 2xh ] × 50 + ( 2 × 3x2) × 100 (2(3xh) + 2xh) × 50 + (2 × 3x2) × 100
    = 8x (3/x2 × 50 + 600x2 
    = 1200/x 600x2 (3)
    2.3 C = 1200/x+ 600x2 = 1200x−1 + 600x2
    dC = − 1200x−2 + 1200x
    dx
    0 = − 1200/x + 1200x
    ∴ 1200x3= 1200
    x3 = 1
    x = 1 (4)
  3. 3.1 s(t) = 5t3 − 65t2 + 200t + 100
    t = 0 Therefore it is 5(0)3 – 65(0)2 + 200(0) + 100 = 100 metres (2)
    3.2 s´(0) = 15t2 − 130t + 200
    s´(4) = 15(4)2 − 130(4) + 200
    = – 80 metres per minute  (3)
    3.3 The height of the car above sea level is decreasing at 80 metres per minute and the car is travelling downwards hence it is a negative rate of change. (2)
    3.4 s´(t) = 15t2 − 130t + 200
    s´´(t) = 30t − 130
    30t = 130
    ∴ t = 130/30
    t = 4.3 (3)
    [26] 

Related:

© www.myexampapers.com. All rights reserved.